Newtonian tides
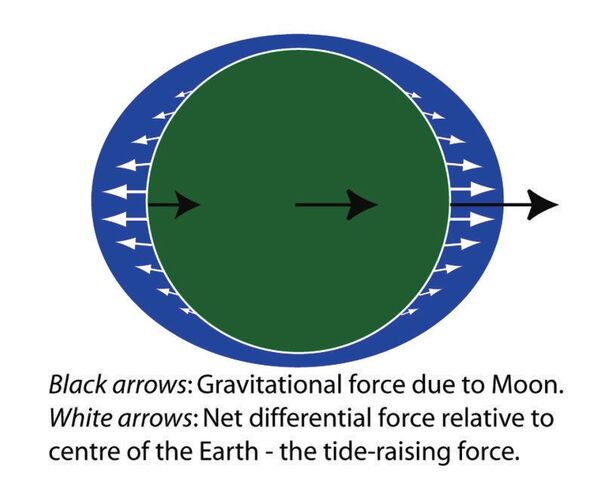
Without involving his mutual gravitation formula, Sir Isaac Newton used sleight of hand methodology to explain the mystery of the high tide on the side of the earth away from the moon......
As distance from the moon increases, the rate of fall towards the moon decreases. Sir Isaac construed this gradient to mean the moon pulls the far side of the earth against the acceleration direction of both the earth and moon inverse square laws and thus stretches the earth across its centre.
The centre of the earth is a natural direction of fall reversal point. Of terrestrial descent, though. Not lunar descent. With this Newtonian diagram lunar descent at the centre of the earth is towards the moon (the middle black arrow). The explanation relies on the middle black arrow becoming a zero direction of fall towards the moon (a zero white arrow) while still existing as the middle black arrow.
The current errant scholastic allowance of the moon's inverse square law actually arithmetically extending to the earth follows............
The current errant scholastic allowance of the moon's inverse square law actually arithmetically extending to the earth follows............
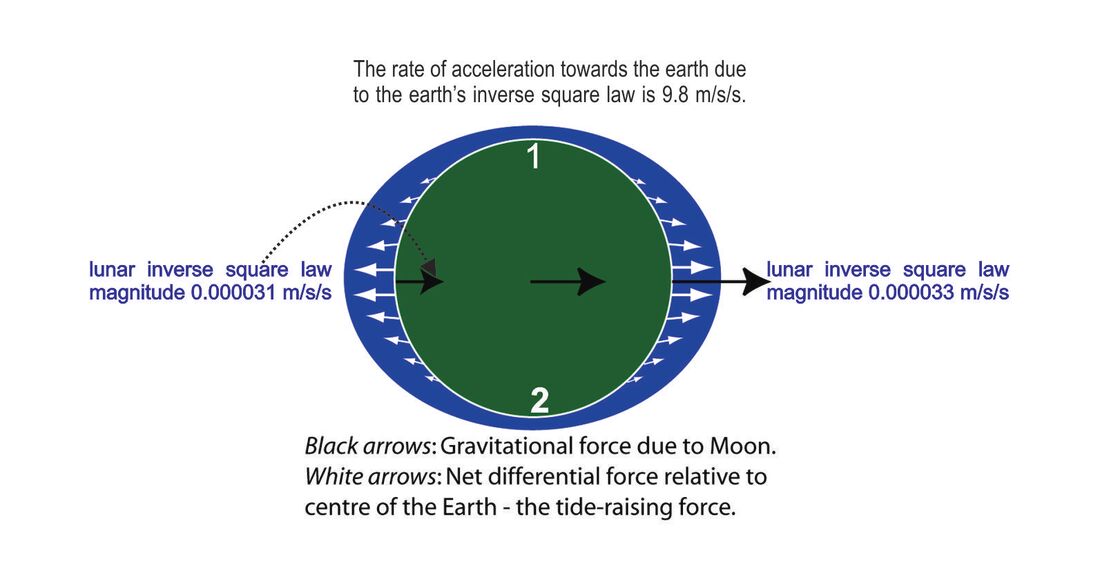
Using the now known lunar surface inverse square law magnitude of 1.6 metre/second/second and basic inverse square law calculations, at the average separation of the earth and moon, the magnitude of the moon's inverse square law at the large black arrow would be 0.000033 m/s/s. And the magnitude of the moon's inverse square law at the small black arrow would be 0.000031 m/s/s.
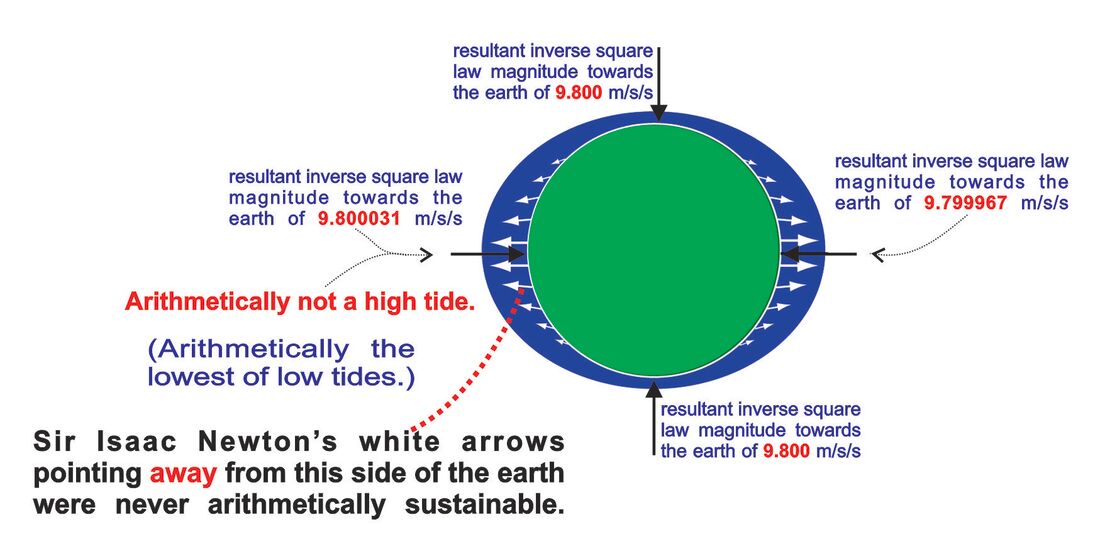
The basic accepted classroom magnitude of this planet's inverse square law at both the small and large black arrows is 9.8 m/s/s. Subtracting the large black arrow from 9.8 m/s/s, the result is a rate of acceleration towards this planet of 9.799967 m/s/s. Except for a few base impossibilities (the smaller inverse square law extension problem and an unintelligible anchor point of the stretch), a high tide under the moon relative to 1 and 2 could have been explained.
At the small black arrow the direction of fall towards the earth and moon is coincidental. On that side the small black arrow magnitude is thus added to this planet's 9.8 m/s/s. Not subtracted. This yields a resultant rate of acceleration of 9.800031 m/s/s directed towards this planet. Which would cause an increase in oceanic weight and result in the lowest of low tides.
The basic accepted classroom magnitude of this planet's inverse square law at both the small and large black arrows is 9.8 m/s/s. Subtracting the large black arrow from 9.8 m/s/s, the result is a rate of acceleration towards this planet of 9.799967 m/s/s. Except for a few base impossibilities (the smaller inverse square law extension problem and an unintelligible anchor point of the stretch), a high tide under the moon relative to 1 and 2 could have been explained.
At the small black arrow the direction of fall towards the earth and moon is coincidental. On that side the small black arrow magnitude is thus added to this planet's 9.8 m/s/s. Not subtracted. This yields a resultant rate of acceleration of 9.800031 m/s/s directed towards this planet. Which would cause an increase in oceanic weight and result in the lowest of low tides.
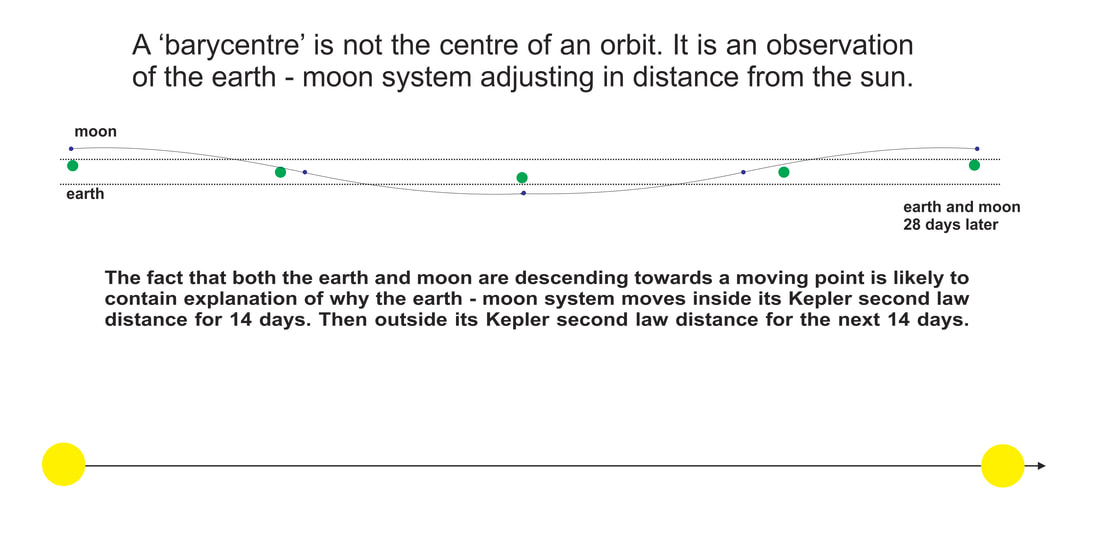
As governments and their educational bureaucracies progressed beyond the life of Sir Isaac, for some his moon stretching the earth reckoning seemed less than the answer to the tides. At the same time astronomers were finding the earth's orbit of the sun to adjust in distance from the sun as the moon orbits the earth. This finding was then added to the Newtonian explanation of the changing ocean height question.
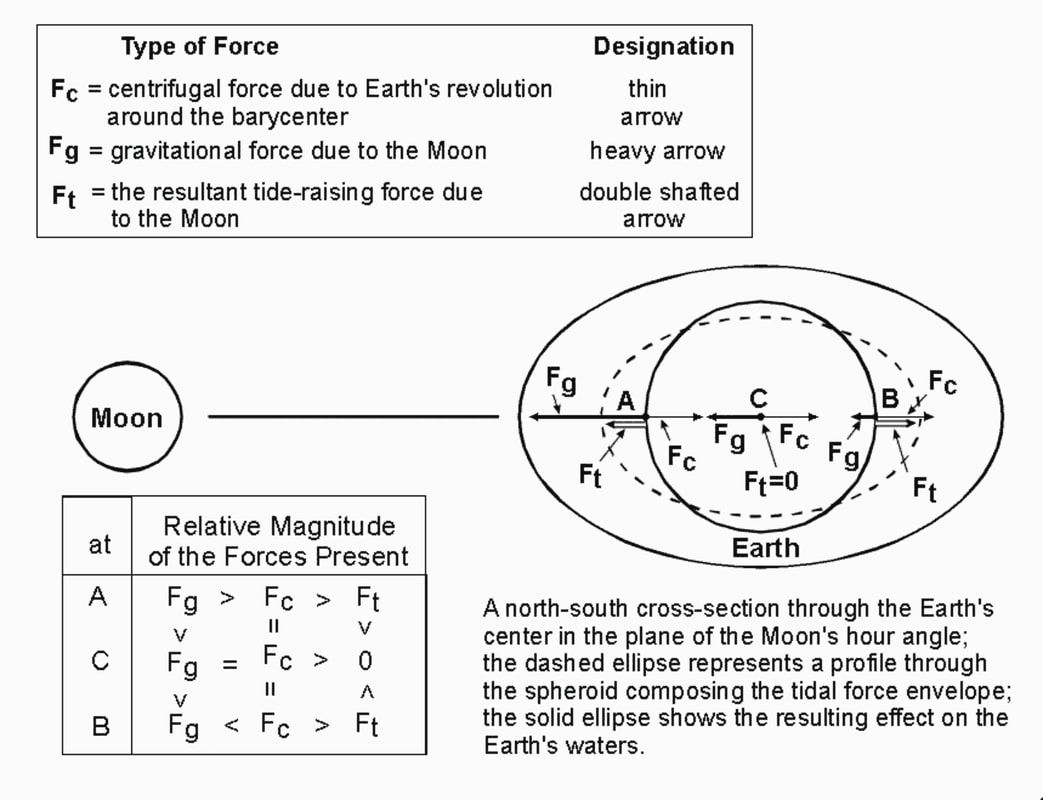
The basis of the addition was the earth and moon are rotating around a joint centre of gravity (a barycentre) that is within the earth and on the moon close side of the earth. On top of the moon's gravity stretching the earth, the earth and its oceans were being thrown away from this barycentre. This added analysis continued within the ad hoc vein of tidally disrespecting the existence of the earth's 9.8 m/s/s surface inverse square law magnitude.
This centrifugal addition did not cause a complete departure from Sir Isaac's original stretch diagram. Including Albert Einstein during his own lifetime, some mathematical physicists were or are still accepting Sir Isaac's lunar stretch of the earth as the completed answer to why one high tide rises against the moon's gravity.
With the barycentre centrifugal force addition to the tides, making this force equal to the magnitude of the moon's inverse square law at the centre of the earth superficially overcame the problem of the moon's inverse square law existing and also not existing at the centre of the earth.
Without mutual gravitation, though, there are no barycentres. For modern earth scientists........
The implication was the earth - moon barycentre is the centre of a gravity field larger than the earth's gravity field.
On this particular diagram, centrifugal force should be increasing in magnitude as the barycentre is moved away from.
There was no articulated tidal correlation with the moon. (An extreme low tide acceleration magnitude on the close side of the moon and an extreme high tide acceleration magnitude on the far side of the moon would be observed if an earth - moon barycentre was part of the earth's tidal explanation.)
There was no explanation of how the joint earth - moon centre constantly relocates as the earth turns on its central axis. Or how a point can be a stable axis of rotation of a composite earth - moon system.
And, of course, from the perspective of modern mathematical earth physics, barycentres did not or would not fit with Einstein's general relativity. If they had of existed barycentres would have been instantaneous occurrences. According to general relativity they occur after the earth and moon have moved from where they were when the centrifugal effect is taking place.
The centrifugal idea was dependent on the existence of a joint mass barycentre centre being the explanation of the adjustment of the earth across its solar orbit as the moon orbits the earth. Mutual gravitation and fixed inverse square law physics were or are secluding the otherwise explanation of smaller adjacent inverse square laws being held apart within the motion of a star's inverse square. That is the gravity fields of the earth and the moon themselves are periodically accelerating and decelerating. The explanation of how so while still maintaining a stable relationship with each other would or should also include why the earth's gravity field moves across its galactic path when the moon's gravity field cuts across that galactic path.
With the barycentre centrifugal force addition to the tides, making this force equal to the magnitude of the moon's inverse square law at the centre of the earth superficially overcame the problem of the moon's inverse square law existing and also not existing at the centre of the earth.
Without mutual gravitation, though, there are no barycentres. For modern earth scientists........
The implication was the earth - moon barycentre is the centre of a gravity field larger than the earth's gravity field.
On this particular diagram, centrifugal force should be increasing in magnitude as the barycentre is moved away from.
There was no articulated tidal correlation with the moon. (An extreme low tide acceleration magnitude on the close side of the moon and an extreme high tide acceleration magnitude on the far side of the moon would be observed if an earth - moon barycentre was part of the earth's tidal explanation.)
There was no explanation of how the joint earth - moon centre constantly relocates as the earth turns on its central axis. Or how a point can be a stable axis of rotation of a composite earth - moon system.
And, of course, from the perspective of modern mathematical earth physics, barycentres did not or would not fit with Einstein's general relativity. If they had of existed barycentres would have been instantaneous occurrences. According to general relativity they occur after the earth and moon have moved from where they were when the centrifugal effect is taking place.
The centrifugal idea was dependent on the existence of a joint mass barycentre centre being the explanation of the adjustment of the earth across its solar orbit as the moon orbits the earth. Mutual gravitation and fixed inverse square law physics were or are secluding the otherwise explanation of smaller adjacent inverse square laws being held apart within the motion of a star's inverse square. That is the gravity fields of the earth and the moon themselves are periodically accelerating and decelerating. The explanation of how so while still maintaining a stable relationship with each other would or should also include why the earth's gravity field moves across its galactic path when the moon's gravity field cuts across that galactic path.
conclusion
When Sir Isaac Newton went into social distancing during his plague, he went off on unhelpful tangents,